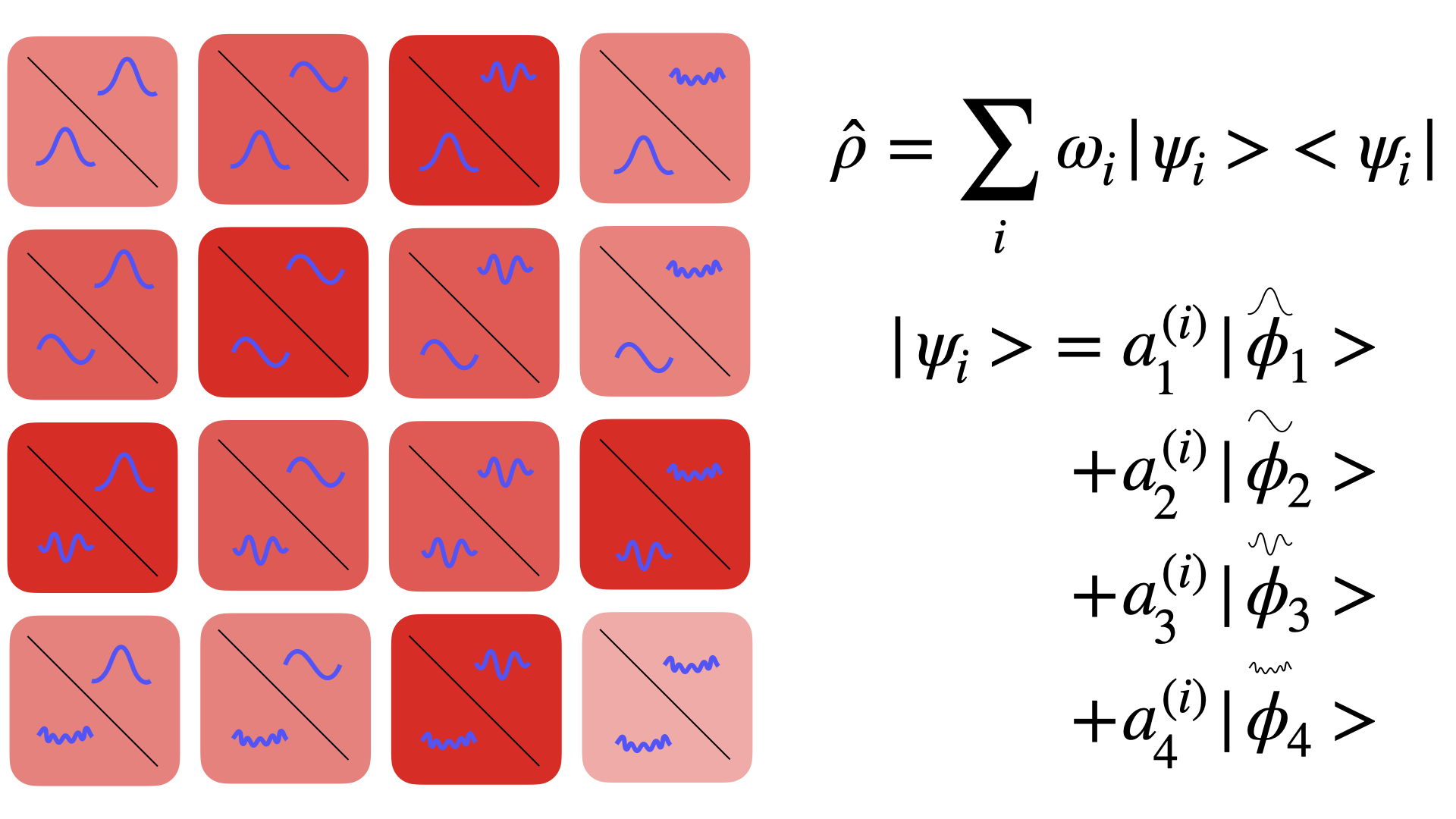
Density matrix: distinguishing pure and mixed states

Let's start by defining density operators for pure states, namely quantum states which can be represented as quantum kets $|\psi>$. In this case, a density operator associated to the quantum state $|\psi>$ is defined as follows \begin{equation} \hat{\rho} \equiv |\psi\rangle \langle \psi| \end{equation} as said, this is a linear operator, and therefore can be represented with a matrix, namely the density matrix. Keeping in mind this distinction, we will utilize the two terms in iterchanghably from now on.
From the definition provided, four properties can be verified for a density operator, namely \begin{align} I)& \hspace{0.5cm} \hat{\rho}^2 = \hat{\rho} \hspace{1cm} \text{$\hat{\rho}$ is a projector}\\ II)& \hspace{0.5cm} \hat{\rho}^{\dagger} = \hat{\rho} \hspace{1cm} \text{$\hat{\rho}$ is Hermitian}\\ III)& \hspace{0.5cm} Tr(\hat{\rho}) = 1 \hspace{1cm} \text{Normalization}\\ IV)& \hspace{0.5cm} \hat{\rho} ≥ 0\hspace{1cm} \text{$\hat{\rho}$ is positive semidefinite} \end{align}

\begin{equation} Tr(\hat{\rho}) = \sum_s \langle s|\hat{\rho}|s\rangle = \sum_s \langle s|\psi\rangle \langle \psi|s\rangle = \sum_s |\langle \psi|s\rangle |^2 = 1 \end{equation} where we have exploited the normalization of $\lvert\psi\rangle$. Finally, to prove property IV, we know that an operator is positive semidefinite if and only if \begin{equation} \forall |s\rangle \hspace{0.5cm} \langle s|\hat{\rho}|s\rangle \hspace{0.2cm} ≥ \hspace{0.2cm} 0 \end{equation} but from the definition of $\hat{\rho}$ \begin{equation} \langle s|\hat{\rho}|s\rangle= |\langle s|\psi\rangle|^2 \end{equation} which is always non-negative, therefore property IV is proved.

Given the operator $\hat{O}$ and the quantum state described by $\hat{\rho}$ the expectacion value of $\hat{O}$ on the state is \begin{equation} \langle \hat{O}\rangle_{\hat{\rho}} = Tr(\hat{\rho}\hat{O}) \end{equation} PROOF \begin{equation} Tr(\hat{\rho}\hat{O}) = \sum_s \langle s|\hat{\rho}\hat{O}|s\rangle = \sum_s \langle s|\psi\rangle \langle \psi|\hat{O}|s\rangle = \sum_s \langle \psi|\hat{O}|s\rangle\langle s|\psi\rangle = \langle \psi|\hat{O}|\psi\rangle \end{equation} where we have used the fact that $\sum_s |s\rangle \langle s| = \hat{I}$.

Let’s start with pure states. As said, a pure state is a quantum state which can be described by a ray in a Hilbert space over the complex numbers. In other words it’s a complex vector (short of the amplitude and phase) and can be written as a quantum ket $|\psi\rangle$.
On the other hand, a mixed state is a state which can't be described with a ket $|\psi\rangle$, but rather by a probabilistic ensamble of pure states $\{p_i, |\psi\rangle_i\}_{i = 1, ..., N}$.
Let's see how these two kinds of states behave differently when measuring some observable over them.
Given a pure state, if we measure the expectation value of some operator $\hat{O}$ over it, in general the result will be a random variable, only due to the probabilistic nature of quantum mechanics.
When measuring the expactation value of some operator on a mixed state, we have two different sources of randomness, namely the probabilistic nature of quantum mechanics (for each pure state in the ensemble) and the probablistic nature of the ensemble itself. We can refer to the first one as quantum randomness and to the second one as classical randomness. We can picure this "double randomness" in the following way: when measuring an observable on a mixed state, first one of the pure states of the ensamble is selected randomly (classical randomness) and then a quantum measurement is done on this pure state, having a random outcome (quantum randomness).
This also means that a pure state is a state for which we have the maximum possible information, a mixed states on the other hand has an additional source of randomness and we have less information about it.

\begin{equation} \hat{\rho}^{pure} = |\psi\rangle \langle \psi| \end{equation} let's now define the density operator for the mixed state $\{p_i, |\psi\rangle_i\}_{i = 1, ..., N}$ as a combination of the density operators corresponding to the pure states making up the mixed state, namely \begin{equation} \hat{\rho}^{mixed} = \sum_i p_i|\psi\rangle_{ii} \langle \psi| \end{equation} Even for mixed states, in this formalism expectation values can be computed easily \begin{equation} \langle\hat{O} \rangle_{\hat{\rho}^{mixed}} = Tr(\hat{\rho}^{mixed} \hat{O}) \end{equation} PROOF \begin{equation} Tr(\hat{\rho}^{mixed} \hat{O}) = Tr\Big(\sum_i p_i|\psi\rangle_{ii} \langle \psi|\hat{O}\Big) = \sum_s \sum_i p_i \langle s|\psi\rangle_{ii} \langle\psi|\hat{O}|s\rangle = \sum_s \sum_i p_i \langle \psi|\hat{O}|s\rangle \langle s|\psi\rangle_{ii} = \sum_i p_i \langle \psi|\hat{O}|\psi\rangle \end{equation} as expected, the expectation value of $\hat{O}$ over the mixed state is given by the weighted sum of the expectation values over the pure states of the ensemble. Furthermore it can be easily verified that properties II, III and IV still hold, therefore this is a legitimate definition for a quantum state.
However property I doesn't hold, in fact \begin{equation} \hat{\rho}^2 = \sum_{ij} p_i p_j |\psi_i\rangle \langle \psi_i|\psi_j\rangle \langle \psi_j| = \sum_{ij} p_i p_j |\psi_i\rangle \delta_{ij} \langle \psi_j| = \sum_i p^2_i |\psi_i\rangle \langle \psi_i| \neq \hat{\rho} \end{equation} this gives us a simple way of identifying mixed states from pure states in the density operator formalism, namely by checking whether or not $\hat{\rho}^2 = \hat{\rho}$.

\begin{equation} |+\rangle = \frac{|0\rangle+ |1\rangle}{\sqrt{2}} \end{equation} Let's take a look at the corresponding density operator \begin{equation} \hat{\rho}^{(A)} = |+\rangle \langle + | = \frac{1}{2}\begin{pmatrix} 1 \\ 1 \end{pmatrix}\begin{pmatrix} 1 & 1 \end{pmatrix}= \frac{1}{2}\begin{pmatrix} 1 & 1\\ 1 & 1 \end{pmatrix} \end{equation} Let's now consider a second apparatus $\textbf{B}$, producing the quantum state $|0\rangle$ with probability $50%$ ant the state $|0\rangle$ with probability $50%$. Namely the mixed state $\{\frac{1}{2}, |0\rangle ; \frac{1}{2}, |1\rangle\}$. According to the definition provided above, we can write its density matrix \begin{equation} \hat{\rho}^{(B)} = \frac{1}{2}|0\rangle \langle 0|+\frac{1}{2}|1\rangle \langle 1| = \frac{1}{2}\begin{pmatrix} 1 & 0 \\ 0 & 0 \end{pmatrix} + \frac{1}{2}\begin{pmatrix} 0 & 0 \\ 0 & 1 \end{pmatrix} = \frac{1}{2}\begin{pmatrix} 1 & 0 \\ 0 & 1 \end{pmatrix} \end{equation}

Let's now make a measurement in the computational basis $\{|0\rangle, |1\rangle\}$ on $\hat{\rho}^{(A)}$ and $\hat{\rho}^{(B)}$.
In both cases, we obtain the states $|0\rangle$ and $|1\rangle$ with $50\%$ probability and there is no way of distinguishing between the two states in this experimet. What happens if we measure in the rotated basis $\{|+\rangle, |-\rangle\}$?
The apparatus A produces $\lvert +\rangle$ states, therefore we will get the same outcome for every copy of the system. The apparatus B produces the state $|0\rangle$ and $|1\rangle$ with $50\%$ probabilities. We can rewrite these two states as \begin{equation} |0\rangle = \frac{|+\rangle + |-\rangle}{2} \end{equation} \begin{equation} |1\rangle = \frac{|+\rangle - |-\rangle}{2} \end{equation} therefore the outcome will be in both cases $|+\rangle$ $50\%$ of the times and $|-\rangle$ $50\%$ of the times. We have seen that measuring in a different basis makes us appreciate the difference between $\hat{\rho}^{(A)}$ and $\hat{\rho}^{(B)}$.
Let's now try to compute the expectation values of $\hat{\rho}^{(A)}$ and $\hat{\rho}^{(B)}$ on two operators to make some practice with the formulas explained above: let's chose $\hat{\sigma}_x$ and $\hat{\sigma}_z$. We have \begin{equation} \langle \hat{\sigma}_x\rangle_{\hat{\rho}^{(A)}} = Tr\Big[ \frac{1}{2}\begin{pmatrix} 1 & 1 \\ 1 & 1 \end{pmatrix} \begin{pmatrix} 0 & 1 \\ 1 & 0 \end{pmatrix} \Big] = \frac{1}{2} Tr \begin{pmatrix} 1 & 1 \\ 1 & 1 \end{pmatrix} = 1 \end{equation} \begin{equation} \langle \hat{\sigma}_x\rangle_{\hat{\rho}^{(B)}} = Tr\Big[ \frac{1}{2}\begin{pmatrix} 1 & 0 \\ 0 & 1 \end{pmatrix} \begin{pmatrix} 0 & 1 \\ 1 & 0 \end{pmatrix} \Big] = \frac{1}{2} Tr \begin{pmatrix} 0 & 1 \\ 1 & 0 \end{pmatrix} = 0 \end{equation} \begin{equation} \langle \hat{\sigma}_z\rangle_{\hat{\rho}^{(A)}} = Tr\Big[\frac{1}{2} \begin{pmatrix} 1 & 1 \\ 1 & 1 \end{pmatrix} \begin{pmatrix} 1 & 0 \\ 0 & -1 \end{pmatrix} \Big] = \frac{1}{2}Tr\begin{pmatrix} 1 & 1 \\ -1 & -1 \end{pmatrix} = 0 \end{equation} \begin{equation} \langle \hat{\sigma}_z\rangle_{\hat{\rho}^{(B)}} = Tr\Big[ \frac{1}{2} \begin{pmatrix} 1 & 0 \\ 0 & 1 \end{pmatrix} \begin{pmatrix} 1 & 0 \\ 0 & -1 \end{pmatrix} \Big] = \frac{1}{2} Tr \begin{pmatrix} 1 & 0 \\ 0 & -1 \end{pmatrix} = 0 \end{equation}